Spiritual and mathematical aspects of seeds

[HamdenRice]

<I am cross posting this from the Religion/Theology Forum because I thought you seed and plant lovers might find it interesting! Enjoy for this seedless time of our lives!>

I was having a conversation with a professor of medicine about this topic. As a bit of explanation, I have to explain that she has a very unusual background. She is both a clinician and a professor of medicine, but her hobby is botany, specifically botanical illustration, and has been studying botany and botanical illustration for years at the New York Botanical Gardens.

She finds seeds and plants to be exceptionally mysterious, almost spiritual, and understands that mystery through mathematics. Because she has to draw and paint almost photo-realistic images of plants, she is meticulously aware of how plants are structured.

The gist of the mystery, as she explained it, is really how so little information in a seed creates such complicated structures. Of course we know a lot about the biochemical processes by which seeds germinate. But we don't know how what seems to be relatively little genetic information creates such massive, complex structures as, say, an oak tree.

The old model was that there was all this information in the genes of the seed that were basically a template for the entire plant, but that seems not to be the case.

Instead, plant genes contain mathematical formulas that are closer to Fibonacci numbers and fractals -- very tiny forumulas that are capable of infinite expansion. At its most simple, a Fibonacci series would be a series in which each number is the sum of the prior two numbers:

0, 1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89,...

Divide any Fibonacci number by the number preceding it, and you get a number that approaches Phi, which is a repeating number that is approximately, 1.61803...

This number has been called the "Golden Ratio."

It turns out that nature in general, but particularly plants (seeds), use Fibonacci numbers to "program" their growth, rather than a complete genetic blueprint. In other words, seeds don't have a genetic plan; they have a formula:

"Count the number of florets spiralling out from the centre of a cauliflower. Look closely and you will find two spirals running in opposite directions, and the number of florets in each are two consecutive Fibonacci numbers."



These same patterns emerge in the spiral of a nautilus, the leaves of a fern, the way leaves branch from vines, and on and on and on in nature. But they also appear in non-organic structures as well. In fact, mathematicians have been able to reproduce natural forms on computers by plotting Fibonacci series and fractals.

Moreover, it turns out that Phi and the Golden Ratio are extremely psychologically pleasing to the human mind, as though like plants, we are pre-programmed to "see" this mathematical formula. It is evident in great paintings, and was developed separately in many of the great art traditions of the world by artists and art teachers who were unaware of the parallel development of its mathematical understanding. Some believe it may explain why certain kinds of nature (an eastern hardwood forest, a high mesa dotted with cactus, for example, which are full of repeating Fibonacci series) provide a kind of spiritual relaxation.

As an avid gardener, I have been especially observant of this phenomenon this past spring and summer -- especially in my tomato plants. I saw that they were not just "growing" but "unfolding" like a Mandelbrot set (see below), with each new small part being a reproduction of the larger part, and with that small part growing larger and creating more smaller parts, and so on -- all from the tiniest of seeds.

In other words, these Fibonacci formulas create "fractal" forms. Fractals are geometric forms that can be broken into parts, such that each part is a smaller representation of the larger whole -- or more intriguingly, a larger whole can be constantly created by reproducing the part. The most famous fractal is the Mandelbroit set, which I urge, urge, urge, everyone interested in the concept of universal forms to look at:

http://en.wikipedia.org/wiki/Beno%C3%AEt_Mandelbrot

Fractals in general can be represented as fairly simple repeating series formulas as well. The Mandelbrot set is very analogous to a Fibonacci series, because it is a repetitive calculation, but in this case it includes complex and imaginary numbers.

When Madelbrot numbers first started being graphed on computers by mathematicians a few decades ago, they found that incredibly complicated forms could be generated -- you could, for example, create these amazing beautiful unfolding forms that mathematically were the size of the galaxy on your home pc. But what was really shocking was that inside these graphed Mandelbrot sets could be found forms in both organic and inorganic nature -- from sea horses to individual snowflakes to spiral galaxies.






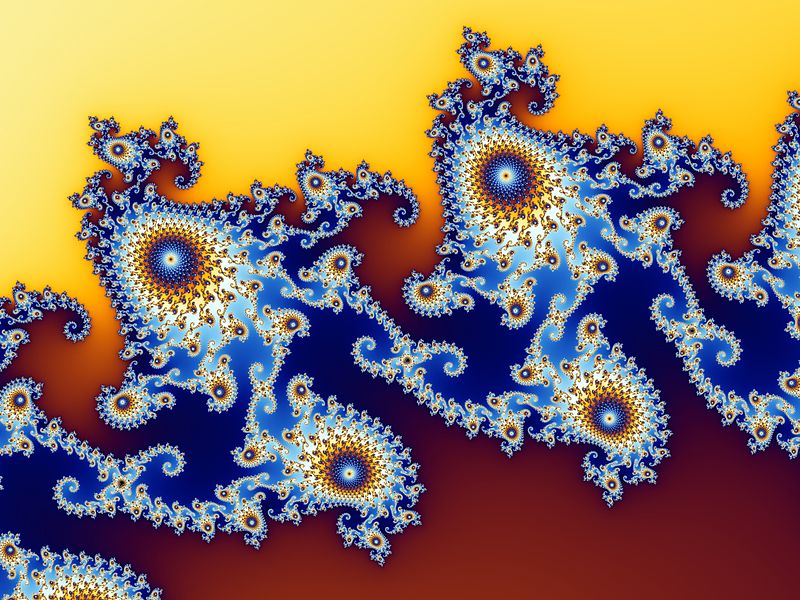
In the emerging field of chaos theory, it was proposed that, for example, both organisms and complex inorganic systems "self organize" using these formulas. As the Wiki article on Mandelbrot puts it:

"He <Mandelbrot> also emphasized the use of fractals as realistic and useful models of many "rough" phenomena in the real world. Natural fractals include the shapes of mountains, coastlines and river basins; the structures of plants, blood vessels and lungs; the clustering of galaxies; and Brownian motion. Fractals are found in human pursuits, such as music, painting, architecture, and stock market prices. Mandelbrot believed that fractals, far from being unnatural, were in many ways more intuitive and natural than the artificially smooth objects of traditional Euclidean geometry:

"Clouds are not spheres, mountains are not cones, coastlines are not circles, and bark is not smooth, nor does lightning travel in a straight line.
—Mandelbrot, in his introduction to The Fractal Geometry of Nature"

<end quote>

These theories really are revolutionizing the way we look at genetics -- especially explaining how genes in seeds (and in us) are not "plans," but unfolding, repeating formulas, like Fibonacci numbers and Mandelbrot sets, and that we share these formulas with other creatures and with the structure of the universe. And it seems that seeing these formulas in nature or in art may be what gives us a sense of beauty, wonder and spiritual fulfillment.

[silverweb]

1. Fascinating and beautiful.

Thank you for posting this.

[Tumbulu]

2. Thanks for this fascinating post!

I appreciate be able to think about it.